User Question: I’m trying to make a parabolic arch, and I THINK my 3 point spline by points is parabolic, but I’m not sure. How can I verify this?
Buildz Von Doom: I have it on good authority that the 3 point spline by points is a true parabola, not an approximation.
User: How do I know you aren’t just a corporate stooge telling me what I want to hear?
BVD: Puny Worm, my word is Law! I have released my corporate flying monkeys to erase you! Before you are consumed by my simian maelstrom, take some small comfort in the parabolic excellence of the 3 point spline by independently verifying it using conic sections. Behold!
Ellipses, hyperbolas, and parabolas are geometrically created using sections of cones. Parabolas [B] are created by cutting a section parallel to the outer surface of the cone. Ellipses [A] are made from sections at a shallower angle than the outer surface, hyperbolas [C] from steeper angles. Often these curves look pretty similar, but they have different characteristics. Parabolas are the most common, often for structural applications.
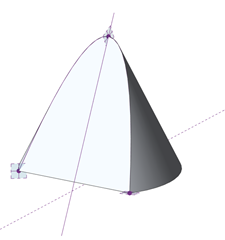
To verify that your three point spline-by-points is in fact a parabola, start with any old cone and draw a reference line aligned with the surface like so:
Draw a void extrusion on the workplane of the reference line and drag it into the cone
Now you have a true parabolic edge on your cut cone. Draw a three point spline by points on it.
Now if you drag your ref line around (the whole line, so that you don’t change the angle) your spline will move with the cut, showing various sizes of parabolic curves.
You can zoom way in on these and see that the curve tracks right on top of the edge, not approximating the parabola.
Download various conic sections from here, .rfa format.
For more on conic sections, check out Wolfram.
Enjoy the monkeys, Insolent Peasant!








Zach,
ReplyDeleteYou're killing me!
Must be a coincidence but I was just toying (unsuccessfully) with catenary arch parametization. Tried Oliver Revit-In-Motion's mathmatical method but couldn't get it to work. Do you know if there's a way to derive catenary arches from solid geometries as you've done with parabolas?
Hmm, I don't know of a way from solid geometries. Can you drive the formula into a family like the one shown here?
ReplyDeletehttp://buildz.blogspot.com/2010/03/formula-driven-curves-and-surfaces.html
I think I have something for this, stay tuned.
is there any way to control the angle of the workplane or reference line base on the intersection of the plan line.
ReplyDeleteSure, you can either put an angular parameter on the ref line that hosts the void element, or just manually pull around the ref line endpoints to change the angel of cut.
ReplyDeleteI know this post is a year old, but I just got tasked in creating a bridge that is parabolic. I've downloaded your cones but what I don't understand is how do you draw the parabola to a set height and then how do you draw the profile so that it is parallel to a section view?
ReplyDeleteLuckily for your application, you don't need the cones. What the cones demonstrate is that the out-of-the-box behavior for a 3 point spline by points is to make a parabolic curve. So, you should just be able to place your 3 points where you need them and get a parabola to build the rest of your bridge on.
ReplyDeleteHi Zach,
ReplyDeletePlease show me how to draw the hyperbola and set parameter for it. Thank you so much!